授课方式： 主讲+互动

教学目的与要求：

1．理解掌握格林公式；

2．理解平面上曲线积分与路径无关的条件、二元函数的全微分求积.

主 要 内 容 ( 按 教 学 大 纲 要 求 )：

重点难点：

1.重点：格林公式、四个等价条件；

2.难点：格林公式、四个等价条件.

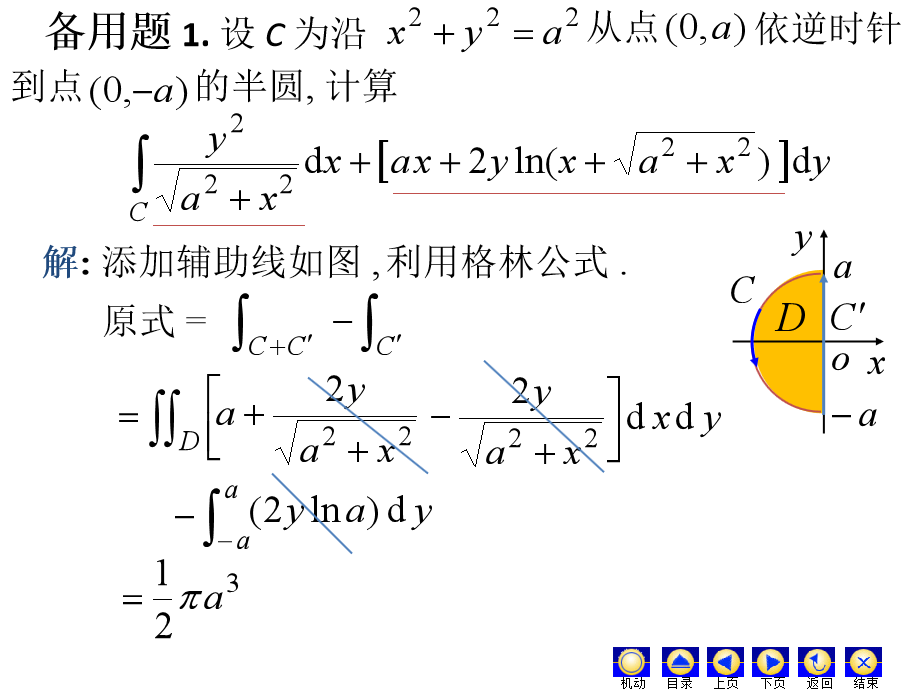
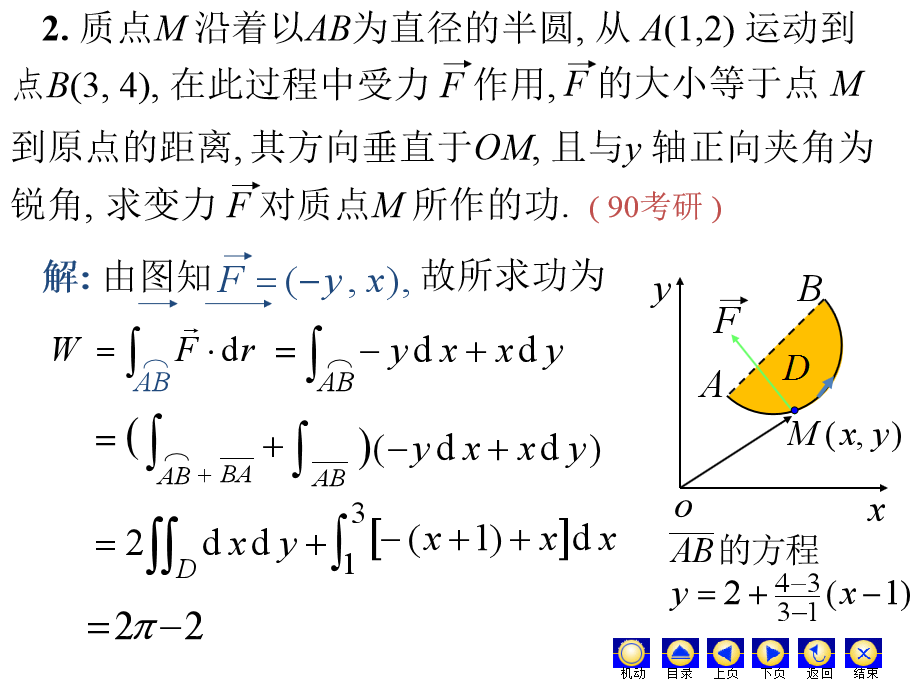
外语词汇：

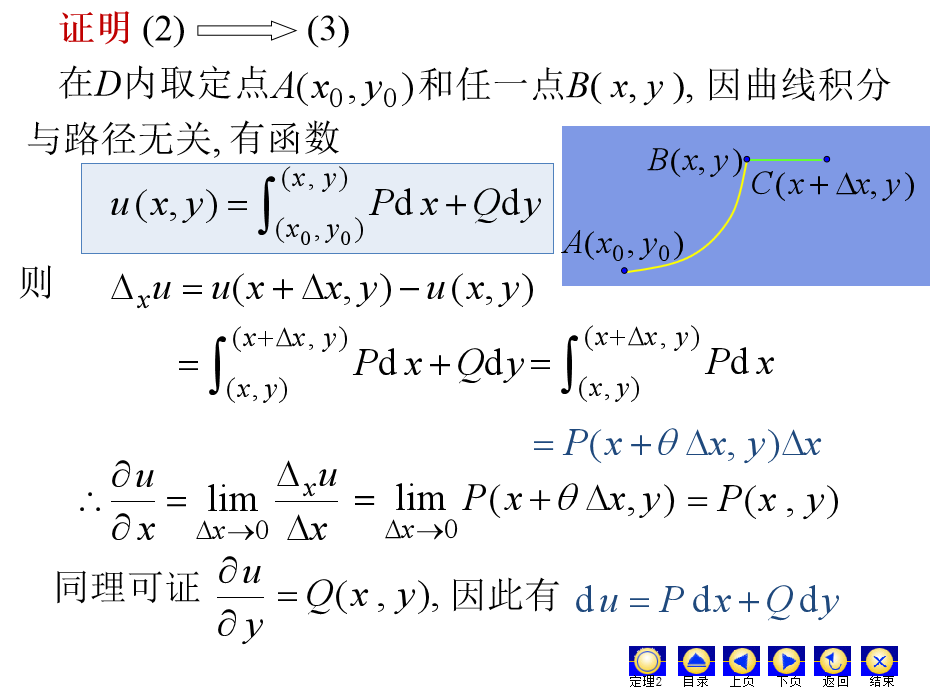
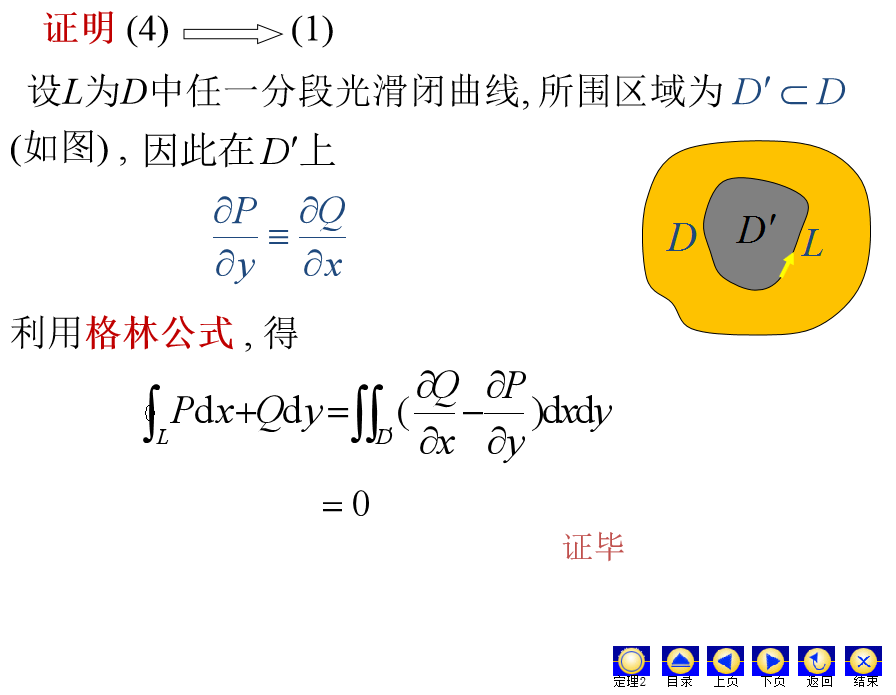
Theorem2  Let G be a simple-connected region.   have continuous first partial derivatives on G.. Then the next conditions are equal conditions.

1.The line integral    is independent of path;

2.The line integral ,where C is an arbitrary piecewise smooth closed curve in the G;

3. , all ( x , y) in G;

4.  is a total differential of some function of two variables.

复习思考题、课堂测试题、课外作业：

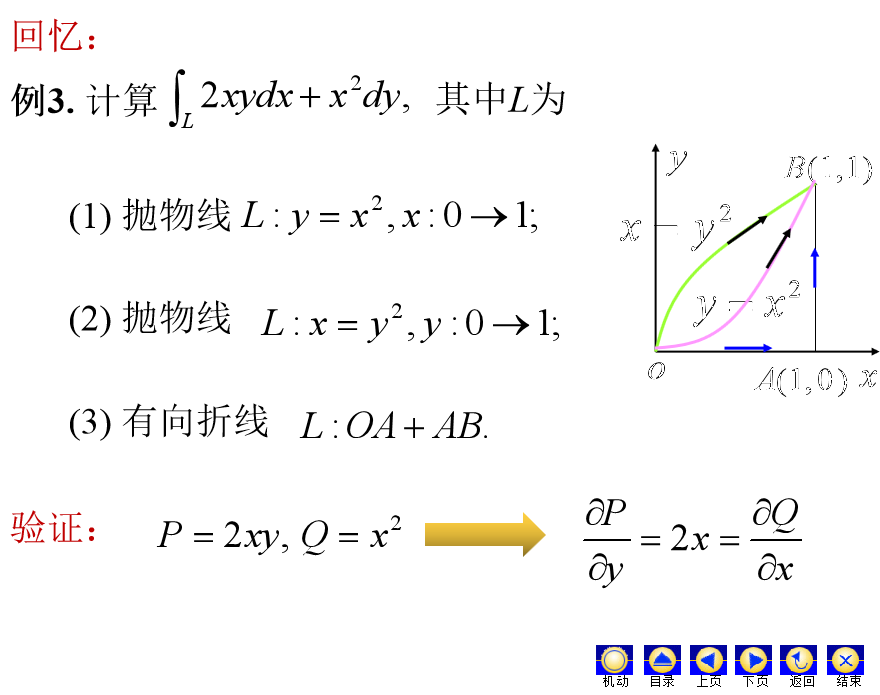
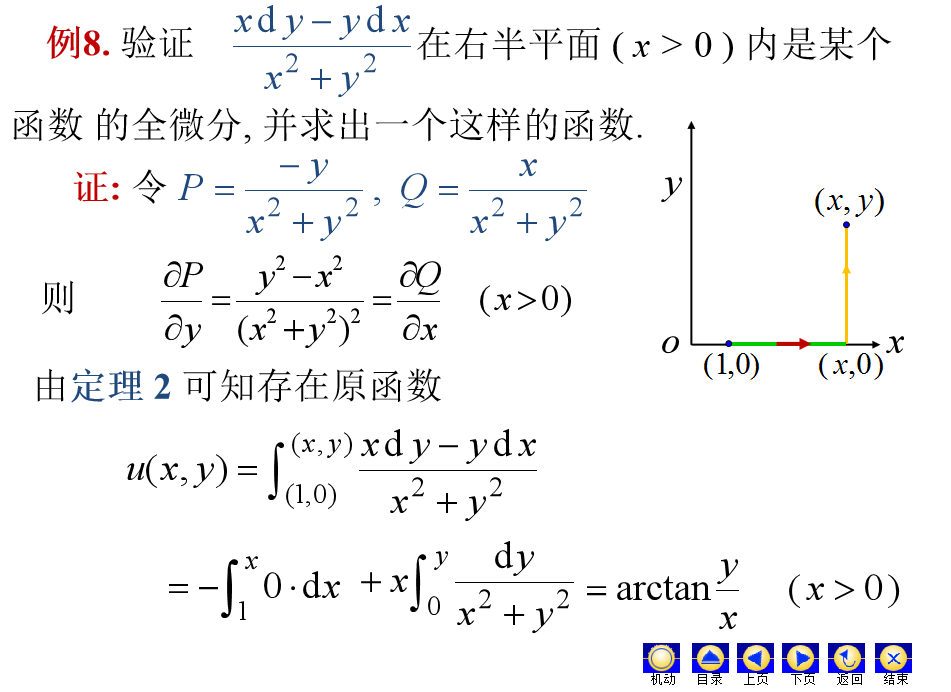
习题11--3   ：3；4（3）；5（1）、（3）、（4）；6（4）、（5）；7