授课方式： 主讲+互动

教学目的与要求：

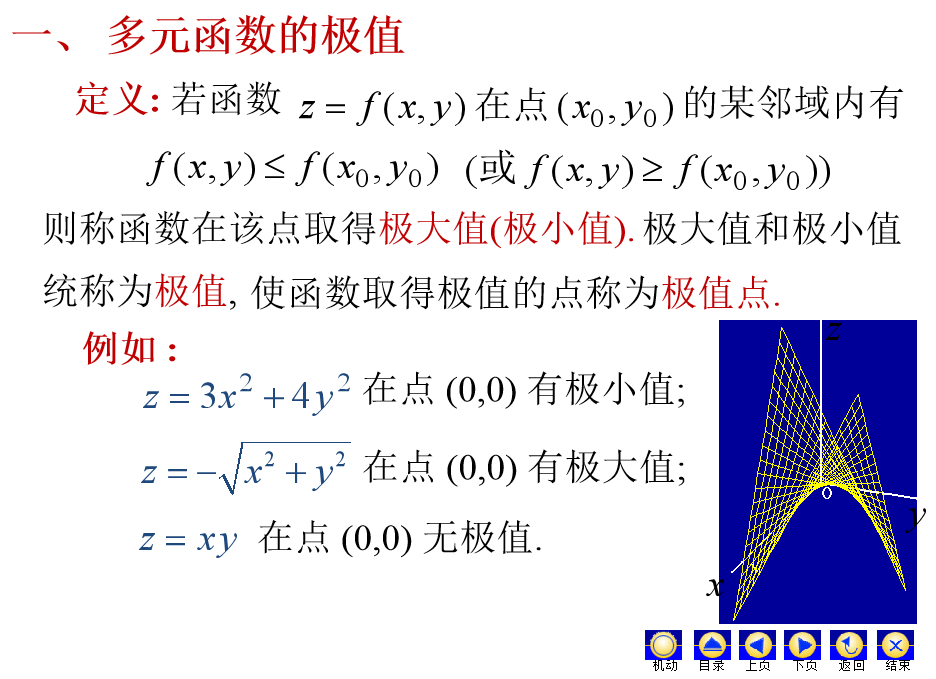
1． 理解多元函数极值和最值的概念；

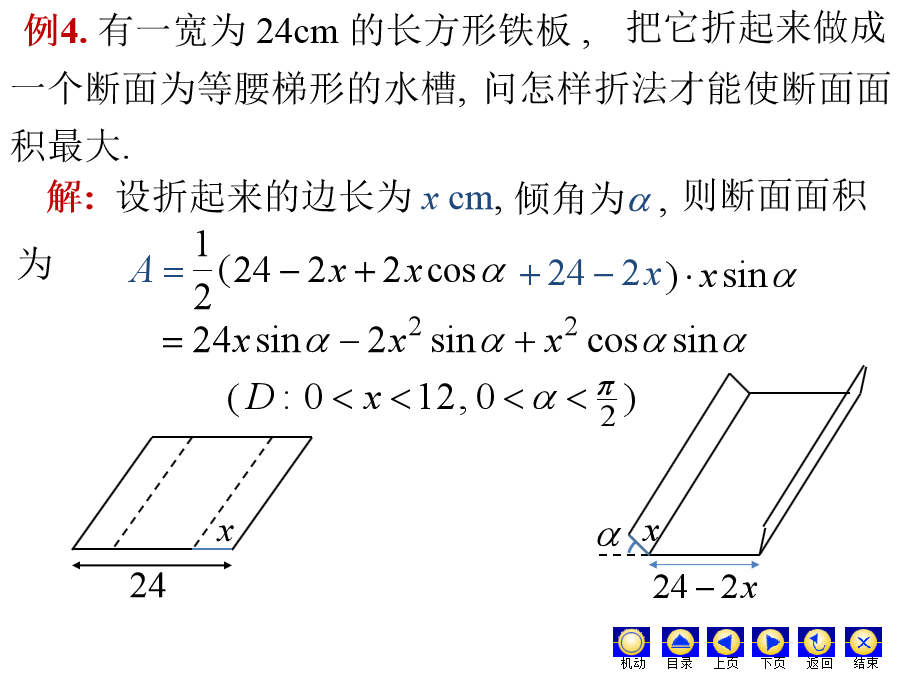
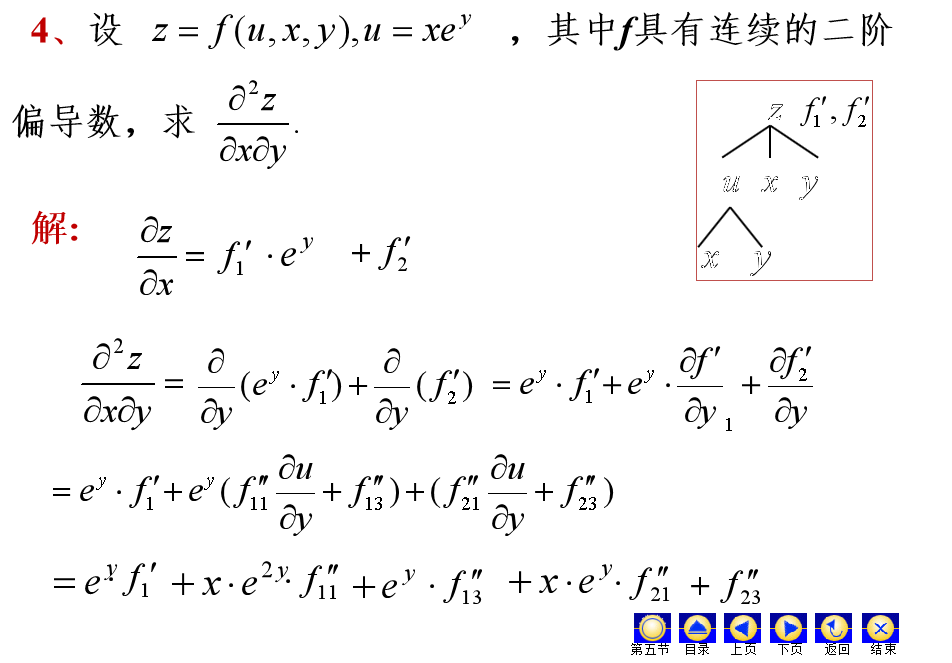
2． 理解掌握多元函数极值存在的必要、充分条件，求法及应用；

3．理解多元函数条件极值的概念、拉格朗日乘数法及应用.

主 要 内 容 ( 按 教 学 大 纲 要 求 )：

重点难点：

1.重点：多元函数极值存在的必要、充分条件，多元函数条件极值的概念、拉格朗日乘数法及应用.

2.难点：同上.

外语词汇： Theorem 1 (Necessary Condition)  If  has partial derivatives at  , and has an extreme value at  ,then we have  .

Theorem 2 (Sufficient Condition)  Suppose that   has continuous second partial deirvatives in a neighborhood  of   and that  .Let  .Then

(1)if  and  , is a local maximum value;

(2)if  and  , is a local minimum value;

(3)if  , the test is inconclusive.

复习思考题、课堂测试题、课外作业： 习题 9 --9：1；2；3；4题.

推荐课外优秀教学视频：